
I_z = \frac where I_z is the MOI about the axis, m is the mass of the cylinder, and r is the radius. Specifically, the one for a solid cylinder: Let's even look at your list of moments of inertia. Sure, technically, that is a very squat cylinder or a parallelepiped with a very thin depth, but if you use an MOI calculation for a 2-D object with a given mass per unit area, the answer between the 3-D and the 2-D calculations will be negligibly different - implying that the 2-D approximation is very, very good. Consider if I made a square and a circle out of very thin sheet metal.

Genralz, in case you mean MOI of a cylinder or cubiod etc.īut I'm not just "making up a mass that has no meaning". Yes you can make up an mass that has no meaning, I don't care. The simple fact is that the MOI formula requires a mass to have some meaning. To a very large extent, it doesn't really matter at all that no 2-D object has mass, or that there is no such thing as a perfect circle, mathematically such objects do exists and we can perform mathematics on them.įrom what I read genralz isn't interested in math for the math. It is a simplifying assumption made primarily to make the math easier and get a result that is going to be very close to the real world. Just like mathematically we can and do use point masses and point charges all the time, despite there being no such thing in the real world. Mathematically we can assign an area density or mass per unit area and make it mathematically have mass. If you want to start down that road, why not just say that a circle or square can't have an MOI because there is no such thing as a perfect circle or perfect square? Rod Smith, P.E.Kedas, while physically being merely a 2-D object a circle or square wouldn't have any mass in the real world, it doesn't prevent us from mathematically talking about a circle or square's MOI. For that application of load, you'll have bolts in tension, but I believe the compression will typically be carried through contact of the plates (unless there are standoff sleeves around the bolts carrying the compression, of course). For out-of-plane moments (producing tension or prying on the bolts), I think you would typically calculate the moment of inertia about an axis closer to the compression edge of the plate. In my design work, I've never had occasion to need Ix or Iy for a bolt group individually only combined for the polar I to calculate forces due to an in-plane moment (producing shear on the bolts, for web splice plates on an I-beam, e.g.). I wonder if we may have skipped over a more important aspect about how and when to apply the moments of inertia once you have calculated them.
MOMENT OF INERTIA OF A CIRCLE HOW TO
With all of the posts, you should have a pretty good handle on how to calculate Ix and Iy, and the polar I (Ix + Iy, or the equation I posted).

Yes Amar-Dj, you've got a handle on the units. Rod Smith, P.E., The artist formerly known as HotRod10 RE: Moment of Inertia of a bolt group
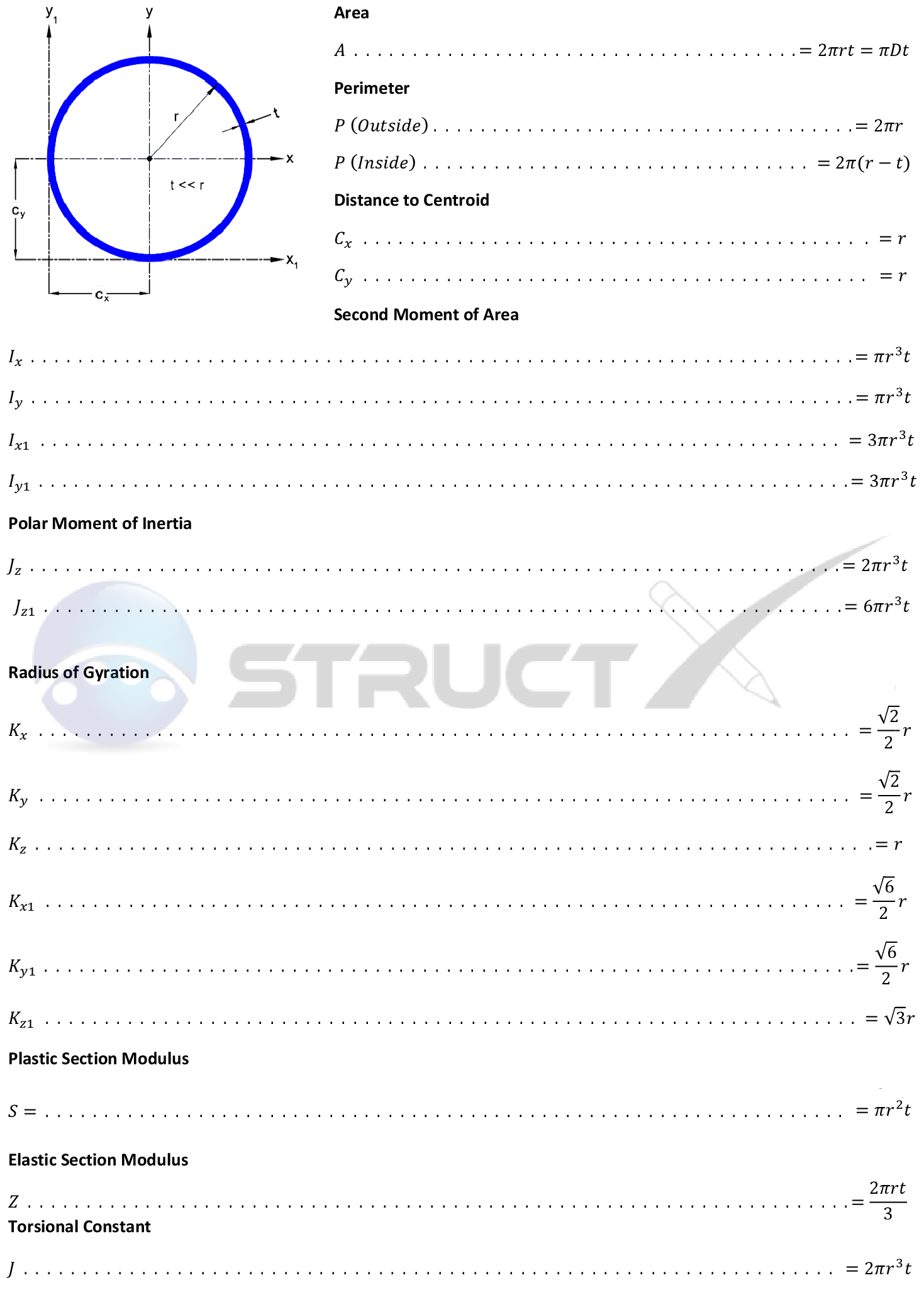
You can add them up individually, or combine and reduce the terms to simplify it for larger groups. I don't have time right now to recreate the derivation, but it should just be a matter of rearranging and combining the equations for Ix and Iy given in the thread I linked to above.Įdit: In it's most basic sense, the polar moment of inertia of a bolt group is the summation of d 2, where d is the distance from the centroid of the group to the center of each bolt. The formula I use for the total polar I of a bolt group (that I either derived or found a long time ago) is: The formula and derivation can be found in this thread. The moment of inertia of the bolts themselves about their individual centroids is ignored as being inconsequential.įor the polar moment of inertia, which is what you would use to calculate the force for a bolt group where a moment is about the centroid of the bolt group, is Ix + Iy. The reason the units are mm 2 is that the "I" of the bolt group only considers the "Ad 2" portion of the moment of Inertia calculation (Io + Ad 2), where "A" is set equal to 1 for convenience of the calculations (so you don't have to multiply by the area of the bolt to get stress and divide it back out to get force).


 0 kommentar(er)
0 kommentar(er)
